2.2 Definition Of Derivativeap Calculus



Derivative of an inverse function: d ( )f x1 ( ) dx − = ( )1 ( ) 1 f f x′ − The derivatives of inverse functions are reciprocals. Rolle’s Theorem: If f is continuous on a, b, differentiable on. The concept of a limit or limiting process, essential to the understanding of calculus, has been around for thousands of years. In fact, early mathematicians used a limiting process to obtain better and better approximations of areas of circles. Calculus is important in all branches of mathematics, science, and engineering, and it is critical to analysis in business and health as well. In this chapter, we explore one of the main tools of calculus, the derivative, and show convenient ways to calculate derivatives.

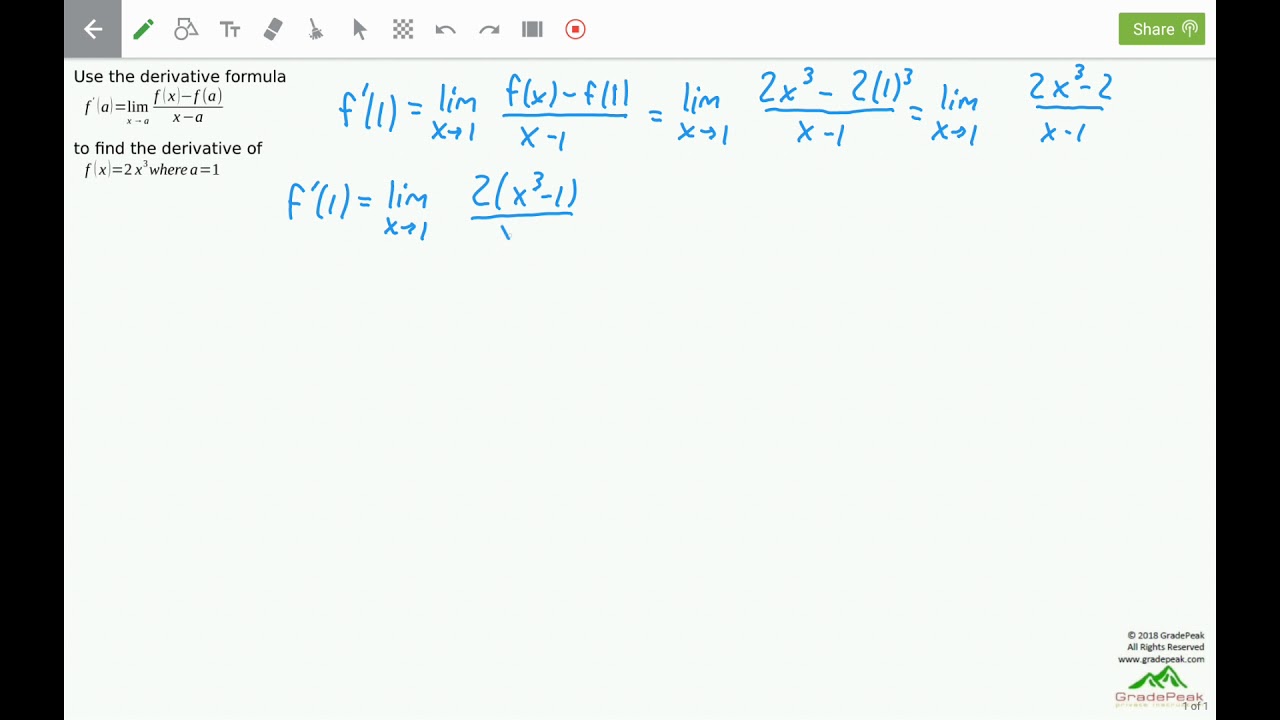
If we recall the definition of a derivative of a function at a point, one of the definitions is. If we compare this definition to the limit we see that that this is the limit definition of a derivative, so we need to find the function and the point at which we are evaluating the derivative at. If you input an x into f prime, you're getting the slope of the tangent line at that point. Now, another notation that you'll see less likely in a calculus class but you might see in a physics class is the notation y with a dot over it, so you could write this is y with a dot over it, which also denotes the derivative. You might also see y prime.

« Previous | Next »

Overview

In this session we apply the main formula for the derivative to the functions 1/x and x^n. We'll also solve a problem using a derivative and give some alternate notations for writing derivatives.

Lecture Video and Notes

Definition

Video Excerpts

Flash and JavaScript are required for this feature.

» Clip 1: Example 1: y=1/x (00:09:00) Happy wheels unblocked games.

» Accompanying Notes (PDF)

From Lecture 1 of 18.01 Single Variable Calculus, Fall 2006

Adventure storywatermelon gaming download. Flash and JavaScript are required for this feature.

Clip 1: Example 1: y=1/x

Flash and JavaScript are required for this feature.

» Clip 2: Harder Problem: Triangles Under the Graph of y=1/x (00:16:00)

» Accompanying Notes (PDF)

From Lecture 1 of 18.01 Single Variable Calculus, Fall 2006

Flash and JavaScript are required for this feature.

Clip 2: Harder Problem: Triangles Under the Graph of y=1/x

Flash and JavaScript are required for this feature.

» Clip 3: Notation for Derivatives (00:02:00)

2.2 Definition Of Derivativeap Calculus Calculator

» Accompanying Notes (PDF)

From Lecture 1 of 18.01 Single Variable Calculus, Fall 2006

Flash and JavaScript are required for this feature.

Clip 3: Notation for Derivatives

Flash and JavaScript are required for this feature.

» Clip 4: Example 2: y=xn (00:08:00)

» Accompanying Notes (PDF)

From Lecture 1 of 18.01 Single Variable Calculus, Fall 2006

2.2 Definition Of Derivativeap Calculus 14th Edition

Flash and JavaScript are required for this feature.

Clip 4: Example 2: y=xn

Worked Example

The Derivative of |x|

« Previous | Next »

Welcome!

This is one of over 2,400 courses on OCW. Explore materials for this course in the pages linked along the left.

2.2 Definition Of Derivativeap Calculus Solver

MIT OpenCourseWare is a free & open publication of material from thousands of MIT courses, covering the entire MIT curriculum.

No enrollment or registration. Freely browse and use OCW materials at your own pace. There's no signup, and no start or end dates.

Knowledge is your reward. Use OCW to guide your own life-long learning, or to teach others. We don't offer credit or certification for using OCW.

Made for sharing. Download files for later. Send to friends and colleagues. Modify, remix, and reuse (just remember to cite OCW as the source.)

Learn more at Get Started with MIT OpenCourseWare